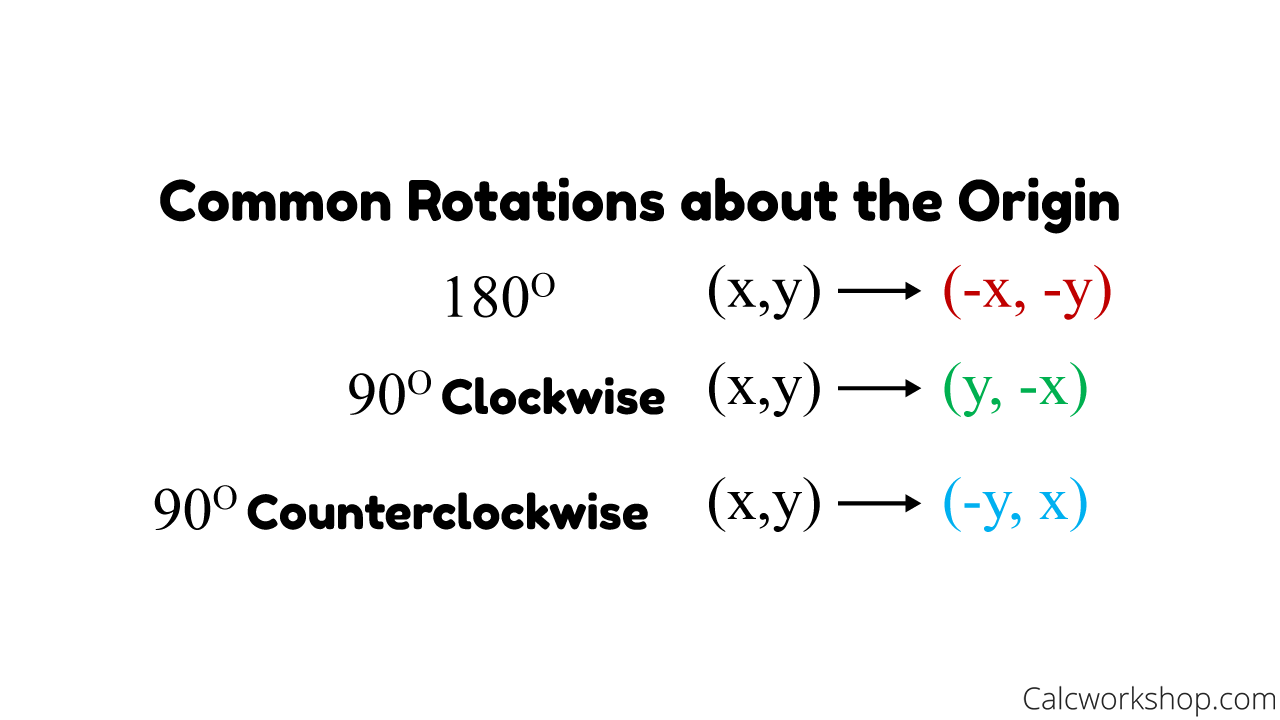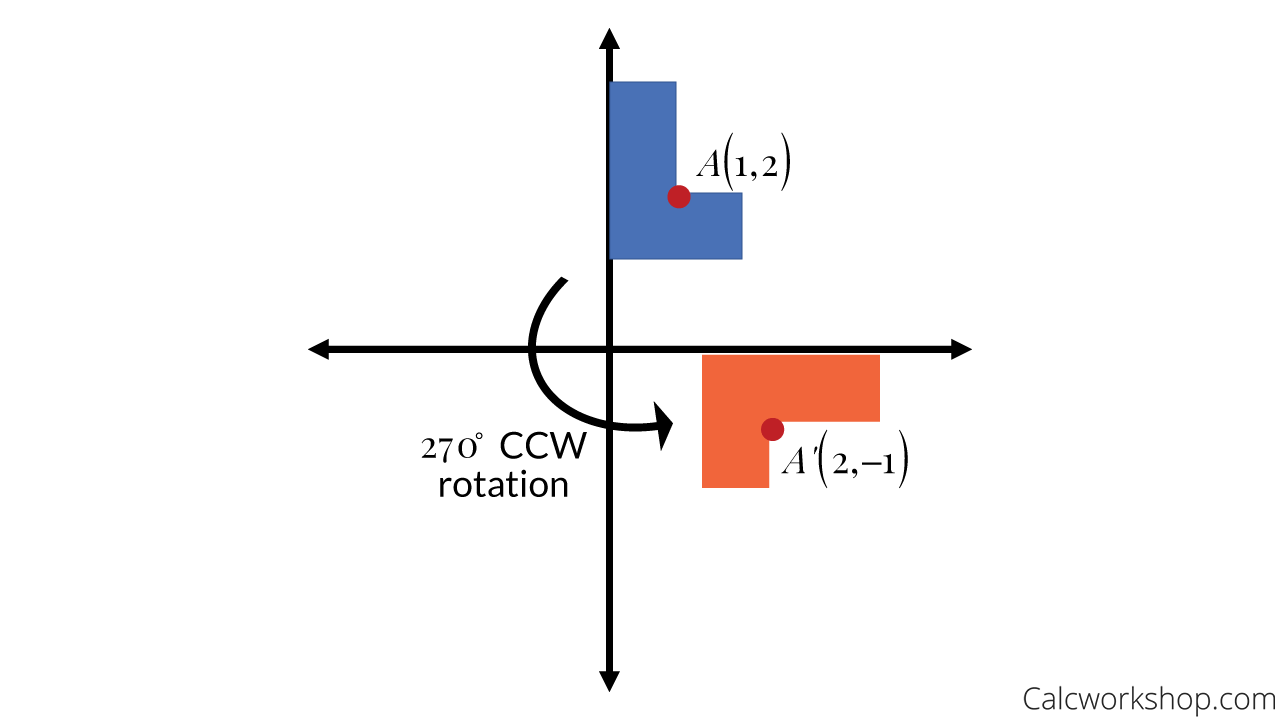Brilliant Strategies Of Info About What Is The Xy Rule For Rotation Line Graph Excel

Rotations intro (article) | khan academy.

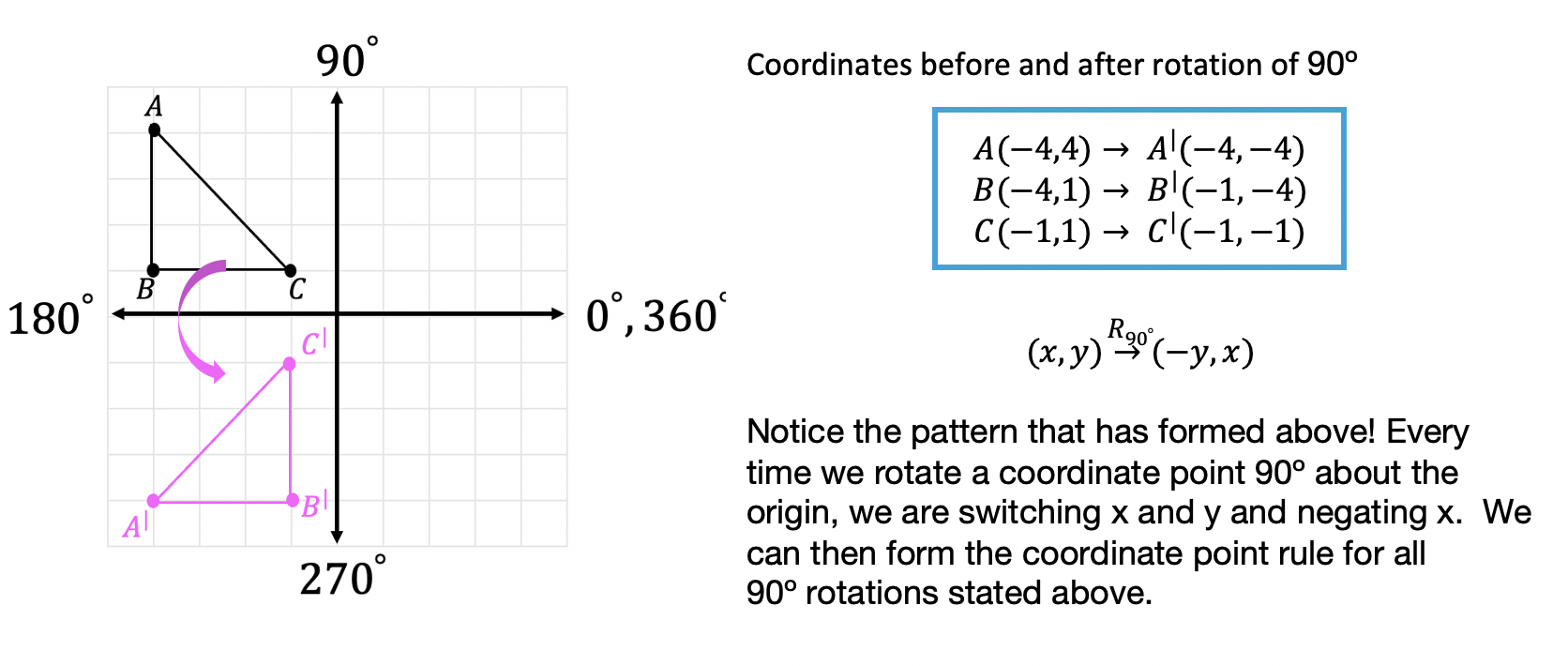
What is the xy rule for rotation. In geometry, rotations make things turn in a cycle around a definite center point. All the rotations around a fixed point that make a group under a structure are called the rotation group of a unique space. This means that the (x,y) coordinates will be completely unchanged!

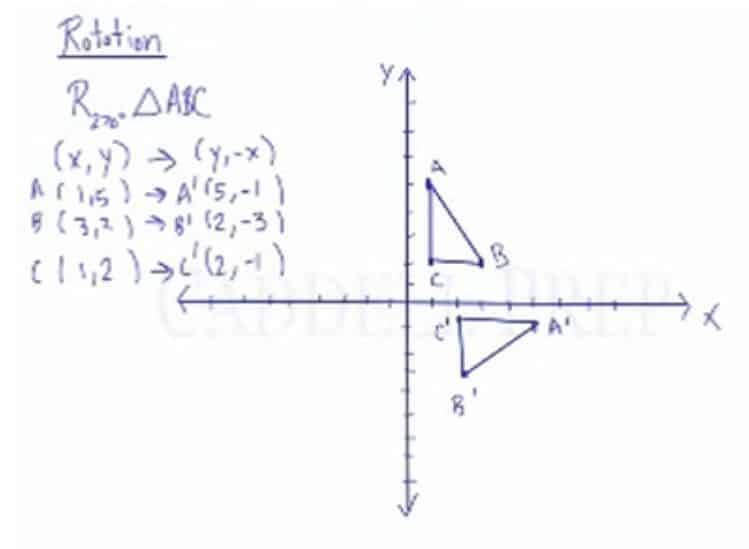
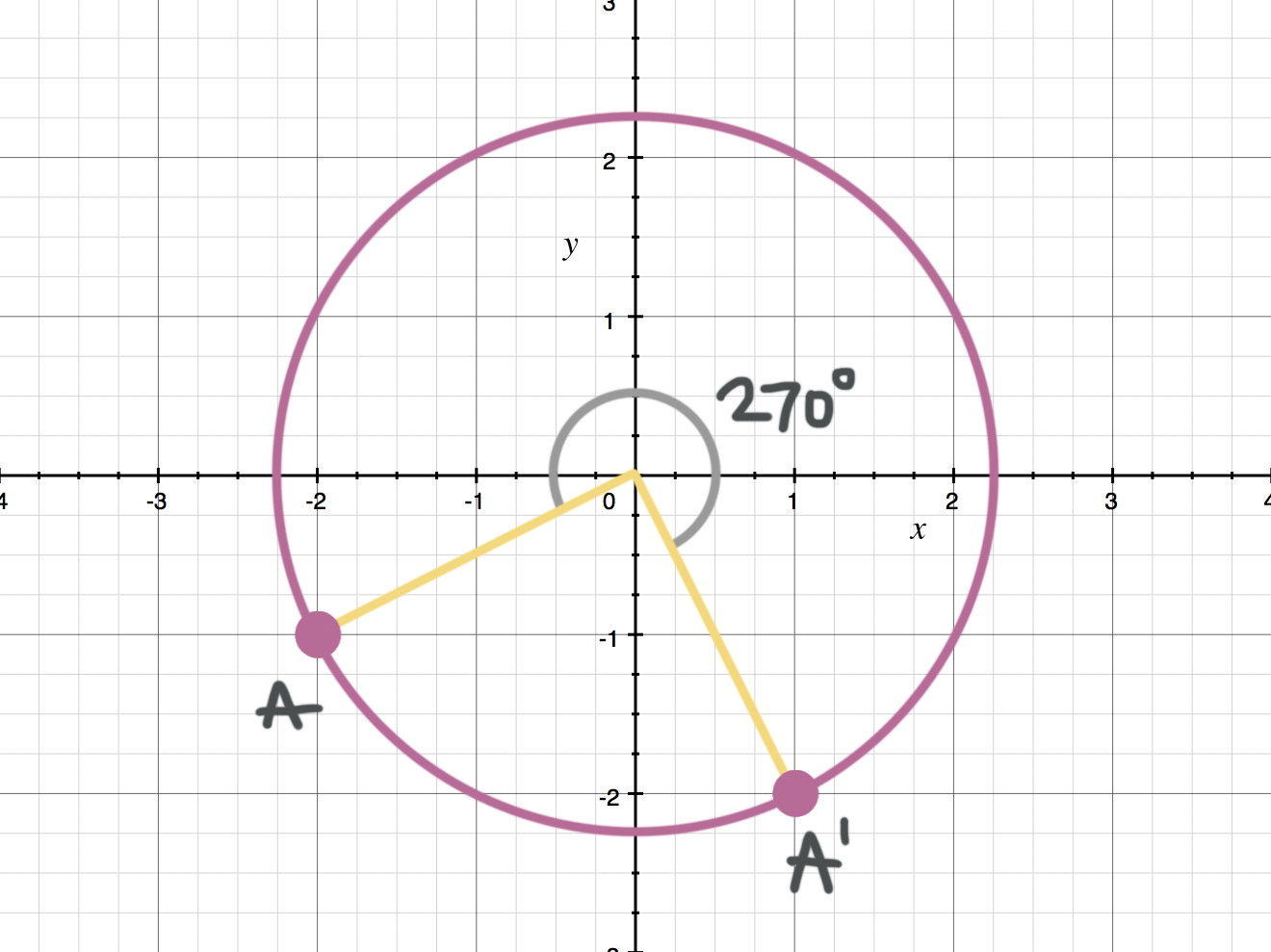
Rotation in mathematics is a concept originating in geometry. A rotation of 270 degrees counterclockwise about the origin is equivalent to the coordinate transformation (𝑥, 𝑦) → (𝑦, − 𝑥). Calculate the instantaneous angular velocity given the angular position function.

Find the angular velocity and angular acceleration in a rotating system. In this free video lesson, i will discuss rotation on the xy coordinate plane. Any rotation is a motion of a certain space that preserves at least one point.

It is possible to rotate different shapes by an angle around the centre point. As long as our motion remains purely rotational, the radial distance r does not change, and we can find the linear velocity by taking the time derivative of \ref{r}: This article focuses on rotations by multiples of ∘ ‍ , both positive (counterclockwise) and.

Review the basics of rotations, and then perform some rotations. So, let's try using these rules to. Find the angular velocity and angular acceleration in a rotating system.

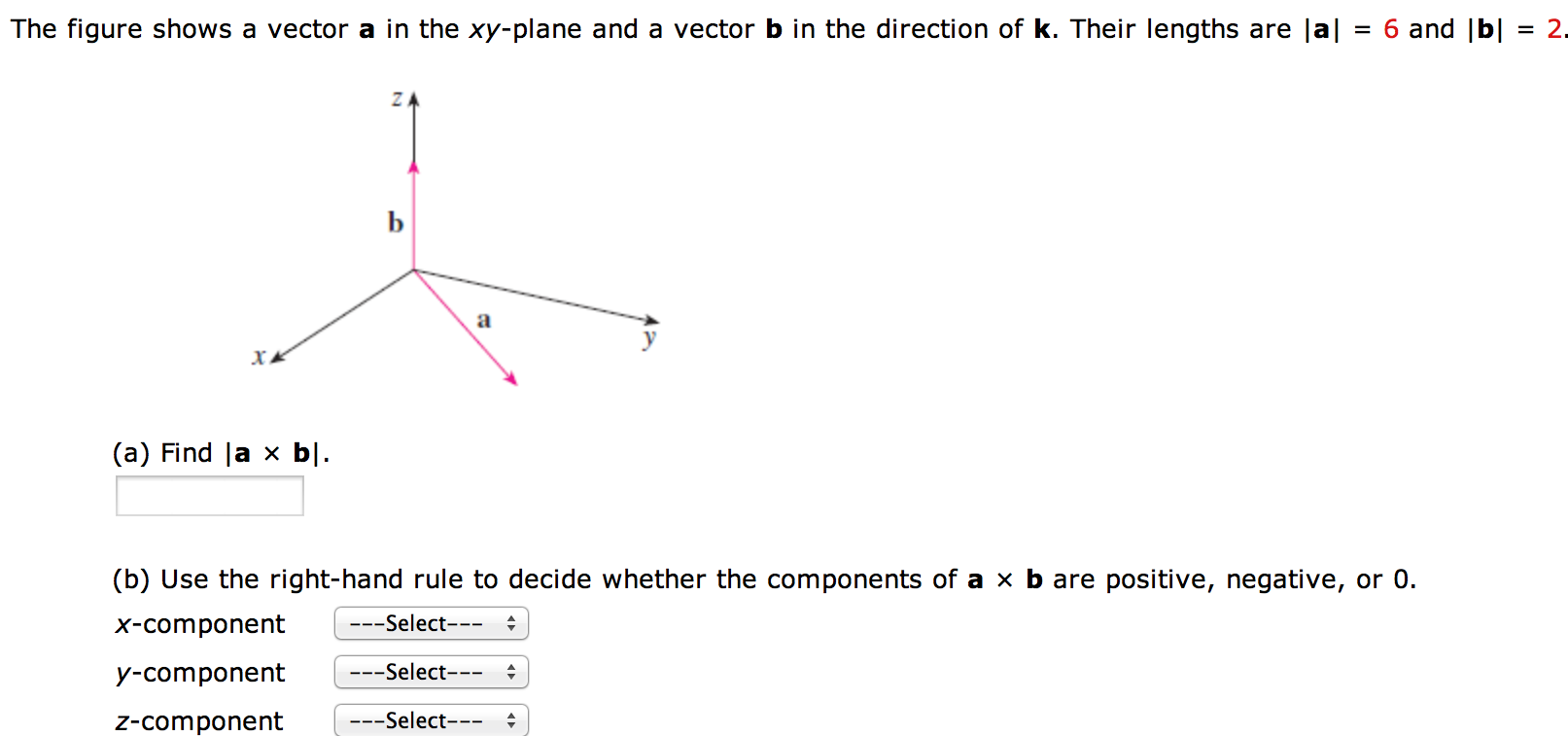
Calculate the instantaneous angular velocity given the angular position function. Rotation means the circular movement of an object around a centre. Explain how angular velocity is related to tangential speed.

To write a rule for this rotation you would write: It can describe, for example, the motion of a. Learn what rotations are and how to perform them in our interactive widget.

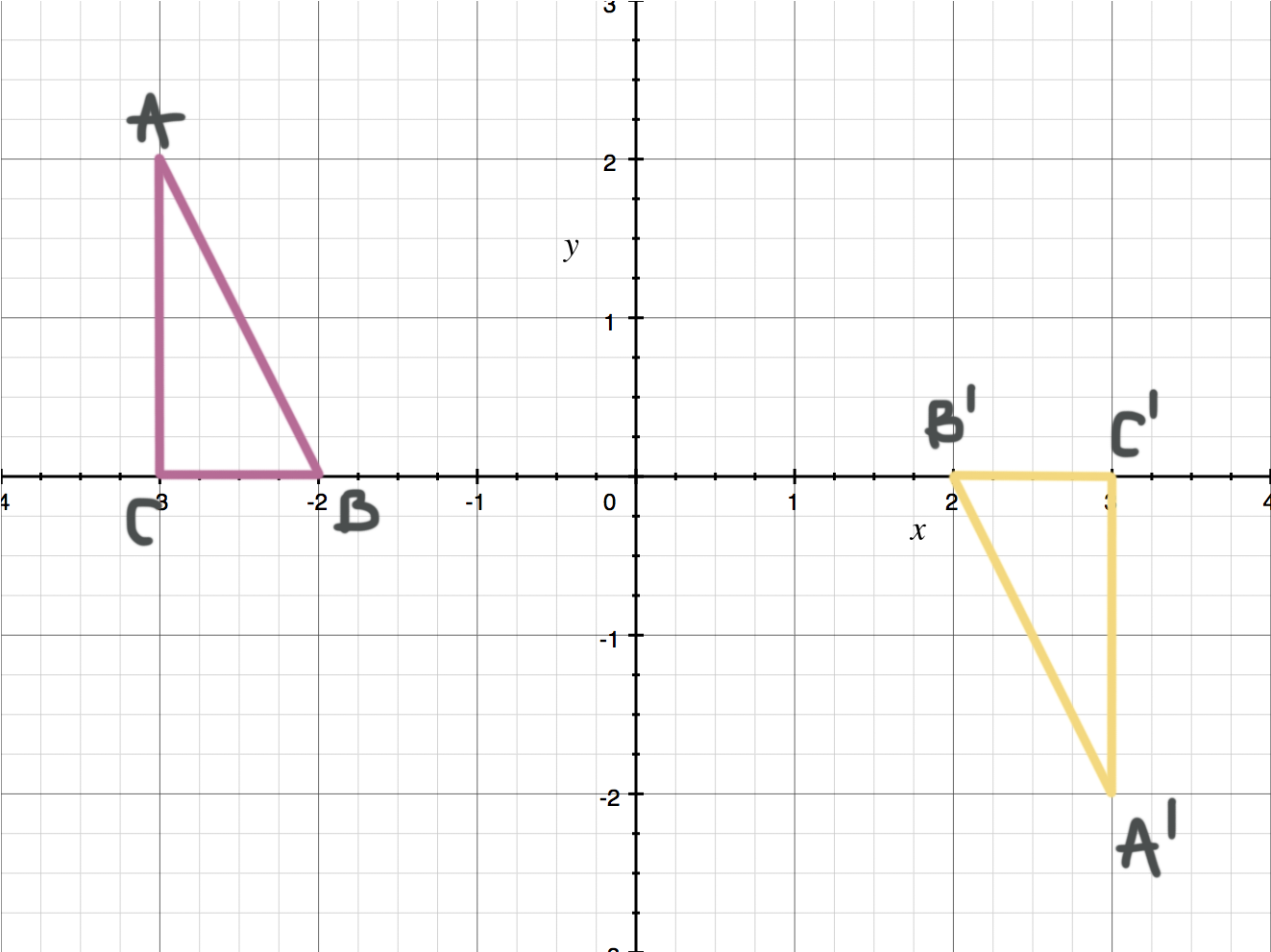
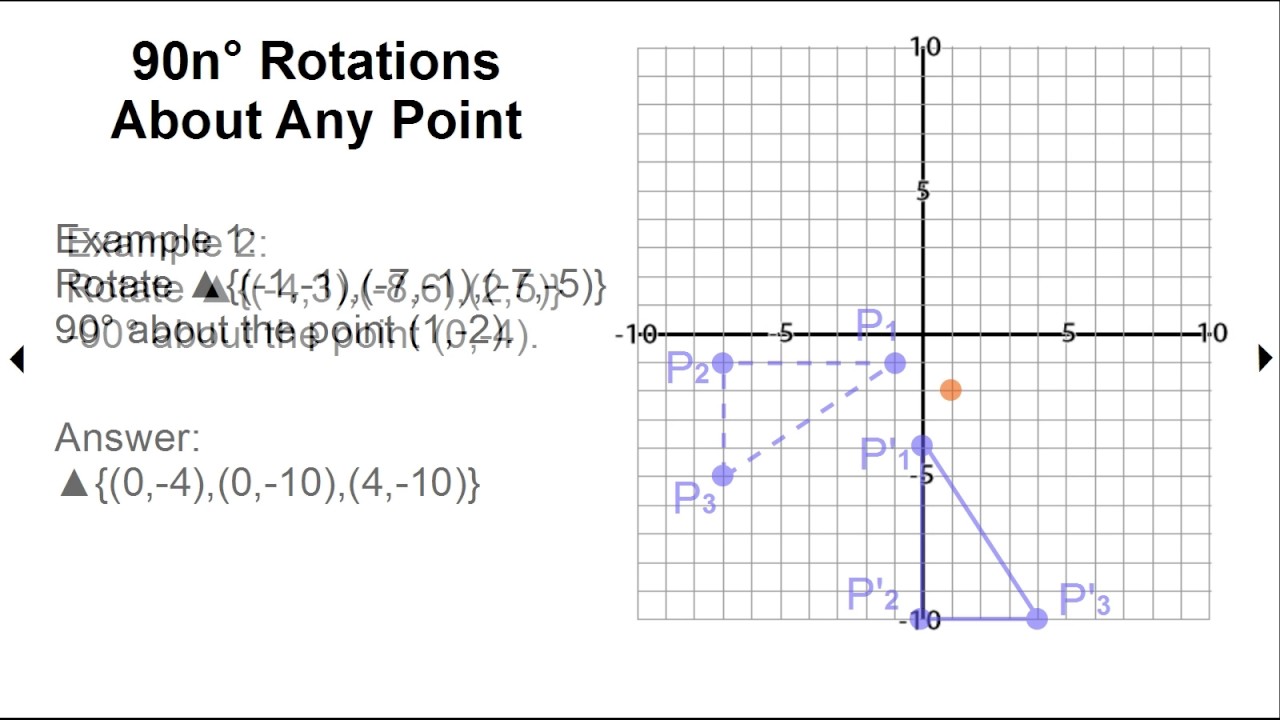
A rotation is an isometric transformation that turns every point of a figure through a specified angle and direction about a fixed point. The rotation formula depends on the type of rotation done to the point with respect to the origin. Example \(\pageindex{}\) thomas describes a rotation as point \(j\) moving from \(j(−2,6)\) to \(j′(6,2)\).

A rotation of 360 degrees about the origin is equivalent to a rotation of 0 degrees and both are equivalent to the coordinate transformation (𝑥, 𝑦) → (𝑥, 𝑦). To describe a rotation, you need three things: Explain how angular velocity is related to tangential speed.

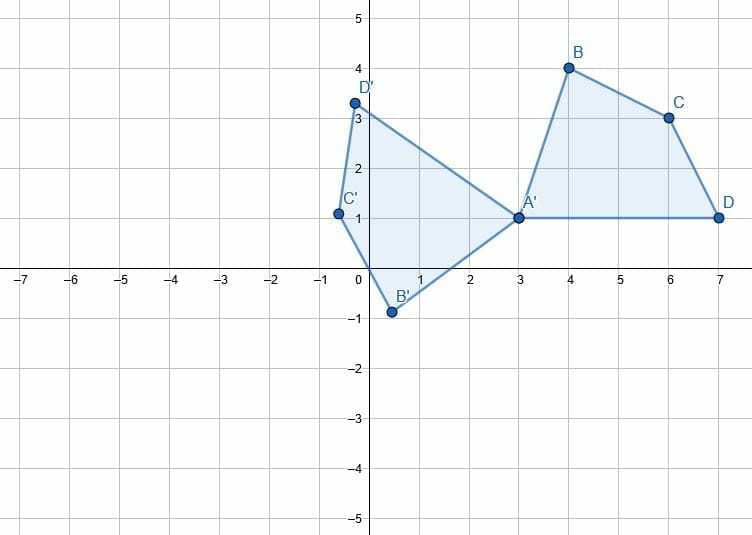
Rotation of an object in two dimensions around a point o. In the figure below, one copy of the trapezoid is rotating around the point. Mathematically speaking, we will learn how to draw the image of a given shape under a given rotation.